Any plane perpendicular to the line of intersection of perpendicular planes intersects them along perpendicular lines.
Sign of perpendicularity of planes
Theorem 1. If a plane passes through a line perpendicular to another plane, then these planes are perpendicular (see figure). 
Theorem 2. If a line lying in one of two perpendicular planes is perpendicular to the line of their intersection, then it is also perpendicular to the second plane (see figure).
Example of application of Theorem 2
Let there be two perpendicular planes and that intersect in a straight line a(see picture). Find the distance from a point A, which lies in the plane and does not lie in the plane, the plane.
In the plane we construct a perpendicular to a through the point A. Let him cross a at the point B. AB- the required distance.
Pay attention to this.
1. Through a point outside the plane, many planes can be drawn perpendicular to this plane (see figure). (But they will all pass through a straight line perpendicular to this plane, which passes through a given point.)
2. If a plane is perpendicular to a given plane, this does not mean that it is perpendicular to an arbitrary straight line parallel to this plane.
For example, in the figure below, and intersect in a straight line b, and a enters one of the planes and . Therefore, straight a at the same time parallel to two perpendicular planes.
Perpendicularity of planes Definition.
Two planes are called perpendicular if the linear angle at the edge of the dihedral angle between these planes is a straight line.
Sign perpendicularity of planes. If a plane passes through a line perpendicular to another plane, then these planes are perpendicular.
Proof. Let a And ? - two intersecting planes, With- the line of their intersection and A- straight perpendicular to the plane?
and lying in a planea. A - point of intersection of linesa And With. In a plane? from point And we will restore perpendicular, and let it be a straight line b. Straight A perpendicular plane? , which means it is perpendicular to any straight line in this plane, that is, straight lines b And Withperpendicular .
Angle between straight lines A And b - linear planes a And ? and it is equal to 90°, so How straight A perpendicular to a straight lineb(proven).By the definition of a planea And ? perpendicular.
Theorem 1.
If from a point belonging to one of two perpendicular planes we draw perpendicular to another plane, then this perpendicular lies entirely in the first plane.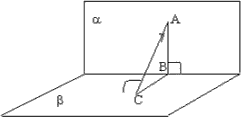 
Proof. Let a And ? - perpendicular planes and With - the straight line of their intersection, A - point lying flat a and not directly belonging to With. Let perpendicular to the plane? drawn from point A does not lie in the plane a, then point C is the base of this perpendicular lies in plane? and does not belong to the line With. From point A we lower the perpendicular AB directly With. Line AB is perpendicularplane (I use Theorem 2).Through straight line AB and point CShall we draw a plane? (a straight line and a point define a plane, and only one). We see that in plane ?
from one point A to straight line BC two perpendiculars are drawn, which cannot happen, which means straight line AC coincides with straight line AB, and straight line AB, in turn, lies completely in the plane a.
Theorem 2.
If in one of two perpendicular planes we draw a perpendicular to their lineintersection, then this perpendicular will be perpendicular to the second plane.
Proof. Let a And ? - two perpendicular planes, With - the line of their intersection and A - straight perpendicular to a straight line With and lying in a planea. A - point of intersection of lines A And With. In plane? from point A we restore the perpendicular, and let it be a straight line b.Angle between straight lines A Andb- linear angle at the edge of the dihedral angle between planes a And ? and it is equal to 90°, since the planea And ? perpendicular. Straight A perpendicular to a straight lineb(according to proven) and direct With by condition. So it's straight A perpendicular to the plane? (
The relation of perpendicularity of planes is considered - one of the most important and most used in the geometry of space and its applications.
From all the variety of mutual arrangements
Two planes deserve special attention and study when the planes are perpendicular to each other (for example, the planes of adjacent walls of a room,
fence and plot of land, door and floor, etc. (Fig. 417, a–c).
The above examples allow us to see one of the main properties of the relationship that we will study - the symmetry of the location of each plane relative to the other. Symmetry is ensured by the fact that the planes seem to be “woven” from perpendiculars. Let's try to clarify these observations.
Let us have a plane α and a straight line c on it (Fig. 418, a). Let us draw through each point of the line c straight lines perpendicular to the plane α. All these lines are parallel to each other (why?) and, based on Problem 1 § 8, form a certain plane β (Fig. 418, b). It is natural to call the plane β perpendicular plane α.
In turn, all lines lying in the plane α and perpendicular to the line c form the plane α and are perpendicular to the plane β (Fig. 418, c). Indeed, if a is an arbitrary line, then it intersects the line c at some point M. A line b perpendicular to α passes through the point M in the plane β, therefore b a. Therefore, a c, a b, therefore a β. Thus, the α plane is perpendicular to the β plane, and the straight line c is the line of their intersection.
Two planes are called perpendicular if each of them is formed by straight lines perpendicular to the second plane and passing through the intersection points of these planes.
The perpendicularity of the planes α and β is indicated by the familiar sign: α β.
One illustration of this definition can be imagined if we consider a fragment of a room in a country house (Fig. 419). In it, the floor and wall are made of boards perpendicular to the wall and floor, respectively. Therefore they are perpendicular. On practice
this means the floor is horizontal and the wall is vertical.
The above definition is difficult to use when actually checking the perpendicularity of planes. But if we carefully analyze the reasoning that led to this definition, we see that the perpendicularity of the planes α and β was ensured by the presence in the β plane of a straight line b perpendicular to the α plane (Fig. 418, c). We came to the criterion of perpendicularity of two planes, which is most often used in practice.
406 Perpendicularity of lines and planes
Theorem 1 (test for perpendicularity of planes).
If one of two planes passes through a line perpendicular to the second plane, then these planes are perpendicular.
Let the plane β pass through a line b perpendicular to the plane α and c - the line of intersection of the planes α and β (Fig. 420, a). All straight lines of the plane β, parallel to the line b and intersecting the line c, together with the straight line b form the plane β. By the theorem about two parallel lines, one of which is perpendicular to the plane (Theorem 1 § 19), all of them, together with the line b, are perpendicular to the plane α. That is, plane β consists of straight lines passing through the line of intersection of planes α and β and perpendicular to plane α (Fig. 420, b).
Now in the plane α, through the point A of the intersection of lines b and c, we draw a line a perpendicular to line c (Fig. 420, c). The straight line a is perpendicular to the plane β, based on the perpendicularity of the line and the plane (a c, by construction, and b, since b α). Repeating the previous arguments, we find that the plane α consists of lines perpendicular to the plane β, passing through the line of intersection of the planes. According to the definition, planes α and β are perpendicular. ■
This feature makes it possible to establish the perpendicularity of the planes or ensure it.
Example 1. Attach the shield to the post so that it is positioned vertically.
If the pillar stands vertically, then it is enough to attach a shield at random to the pillar and secure it (Fig. 421, a). According to the feature discussed above, the plane of the shield will be perpendicular to the surface of the earth. In this case, the problem has an infinite number of solutions.
Perpendicularity of planes |
||
If the pillar stands obliquely to the ground, then it is enough to attach a vertical rail to the pillar (Fig. 421, b), and then attach the shield to both the rail and the pillar. In this case, the position of the shield will be quite definite, since the post and rail define a single plane. ■
In the previous example, the “technical” task was reduced to a mathematical problem about drawing a plane perpendicular to another plane through a given straight line.
Example 2. From vertex A of square ABCD a segment AK is drawn perpendicular to its plane, AB = AK = a.
1) Determine the relative position of the planes AKC and ABD,
AKD and ABK.
2) Construct a plane passing through line BD perpendicular to plane ABC.
3) Draw a plane perpendicular to the plane KAC through the middle F of the segment KC.
4) Find the area of triangle BDF.
Let’s construct a drawing that corresponds to the conditions of the example (Fig. 422).
1) Planes AKC and ABD are perpendicular, according to the condition of perpendicularity of planes (Theorem 1): AK ABD , according to the condition. Planes AKD and ABK are also perpendicular
are polar, based on the perpendicularity of the planes (Theorem 1). Indeed, the line AB through which the plane ABK passes is perpendicular to the plane AKD, according to the sign of perpendicularity of the line and the plane (Theorem 1 § 18): AB AD are like adjacent sides of a square; AB AK , since
AK ABD.
2) Based on the perpendicularity of the planes, for the desired construction it is enough to draw a straight line BD through some points
408 Perpendicularity of lines and planes
line perpendicular to plane ABC. And to do this, it is enough to draw a line parallel to straight line AK through this point.
Indeed, by condition, the line AK is perpendicular to the plane ABC and therefore, according to the theorem about two parallel lines,
our, one of which is perpendicular to the plane (Theorem 1§19), |
|||||||||||||||||
the constructed straight line will be perpendicular to plane ABC. |
|||||||||||||||||
Construction. |
Through the point |
B we conduct |
|||||||||||||||
BE, |
parallel |
||||||||||||||||
(Fig. 423). The plane BDE is the desired one. |
|||||||||||||||||
3) Let F be the midpoint of the segment KC. Pro- |
|||||||||||||||||
we lead through the point |
perpendicular- |
||||||||||||||||
plane |
This straight line |
||||||||||||||||
children direct |
FO, where |
O - center of the square |
|||||||||||||||
ABCD (Fig. 424). Indeed, FO || A.K. |
|||||||||||||||||
like average |
triangle line |
||||||||||||||||
Because the |
perpendicular- |
||||||||||||||||
on surface |
direct FO |
boo- |
|||||||||||||||
det is perpendicular to it, according to the theorem about |
|||||||||||||||||
two parallel lines, one of which |
|||||||||||||||||
ry perpendicular to the plane (Theorem 1 |
|||||||||||||||||
§ 19). That's why |
FO DB. And since AC DB, then DB AOF (or |
||||||||||||||||
KAC). Plane |
BDF passes through a line perpendicular to |
||||||||||||||||
plane KAC, that is, it is the desired one. |
|||||||||||||||||
4) In a triangle |
BDF segment FO |
Height drawn to |
|||||||||||||||
side BD (see Fig. 424). We have: BD = |
2 a as the diagonal of the quad- |
||||||||||||||||
rata; FO = 1 |
AK = |
1 a, by the property of the midline of a triangle. |
|||||||||||||||
Thus, S = 2 BD FO = |
2 2 a |
2 a = |
. ■ |
||||||||||||||
Answer: 4) |
a 2 . |
||||||||||||||||
Study of the properties of the perpendicular- |
|||||||||||||||||
of planes and its applications, let’s start with the simplest |
|||||||||||||||||
that, but very useful theorem. |
|||||||||||||||||
Theorem 2 (about the perpendicular to the line of intersection of perpendicular planes).
If two planes are perpendicular, then a straight line belonging to one plane and perpendicular to the intersection of these planes is perpendicular to the second plane.
Let perpendicular planes
α and β intersect along straight line c, and straight line b in plane β is perpendicular to straight line c and intersects it at point B (Fig. 425). By definition
dividing the perpendicularity of the planes, in the β plane a straight line passes through point B
b 1, perpendicular to the plane α. It is clear that it is perpendicular to the line c. But what-
If you cut a point on a straight line in a plane, you can draw only one straight line perpendicular to the given straight line. That's why
lines b and b 1 coincide. This means that a straight line of one plane, perpendicular to the line of intersection of two perpendicular planes, is perpendicular to the second plane. ■
Let us apply the considered theorem to justify another sign of the perpendicularity of planes, which is important from the point of view of the subsequent study of the relative position of two planes.
Let the planes α and β be perpendicular, straight line c is the line of their intersection. Through an arbitrary point A we draw a straight line c
in planes α and β, straight lines a and b, perpendicular to straight line c (Fig. 426). According to theory
Me 2, straight lines a and b are perpendicular to the planes β and α, respectively, so they are perpendicular to each other: a b . Straight
a and b define a certain plane γ. Line of intersection with planes α and β
perpendicular to the plane γ, based on the perpendicularity of the line and the plane (Theorem 1 § 18): c a, c b, a γ, b γ. If we take into account the arbitrariness of the choice of point A on line c and the fact that through point A of line c there passes a single plane perpendicular to it, then we can draw the following conclusion.
Theorem 3 (about the plane perpendicular to the line of intersection of perpendicular planes).
A plane perpendicular to the line of intersection of two perpendicular planes intersects these planes along perpendicular straight lines.
Thus, another property of perpendicular planes has been established. This property is characteristic, that is, if it is true for some two planes, then the planes are perpendicular to each other. We have one more sign of perpendicularity of planes.
Theorem 4 (second criterion for the perpendicularity of planes).
If the direct intersections of two planes by a third plane perpendicular to the line of their intersection are perpendicular, then these planes are also perpendicular.
Let the planes α and β intersect along a straight line with, and the plane γ, perpendicular to the line with, intersects the planes α and β corresponding
respectively along straight lines a and b (Fig. 427). By condition, a b . Since γ c, then a c. And therefore the line a is perpendicular to the plane β, according to the sign that the line and the plane are perpendicular (Theorem 1 § 18). That's it-
Yes, it follows that the planes α and β are perpendicular, according to the sign of perpendicularity of planes (Theorem 1). ■
Also worthy of attention are theorems about the connections between the perpendicularity of two planes of a third plane and their mutual position.
Theorem 5 (about the line of intersection of two planes perpendicular to the third plane).
If two planes perpendicular to a third plane intersect, then the line of their intersection is perpendicular to this plane.
Let the planes α and β, perpendicular to the plane γ, intersect along the straight line a (a || γ), and A is the point of intersection of the straight line a with
Perpendicularity of planes |
|
plane γ (Fig. 428). Point A belongs to |
|
lives along the intersection lines of the planes γ and α, γ |
|
and β, and, by condition, α γ and β γ. Therefore, according to |
|
determining the perpendicularity of the plane |
|
tey, through point A you can draw straight lines, |
|
lying in the α planes |
and β and perpendicular |
polar planes γ. Because through the point |
|
it is possible to draw only one straight line, per- |
|
perpendicular to the plane, then the constructed |
|
straight lines coincide and coincide with the line |
|
intersections of planes α and β. Thus, straight a is a line |
|
the intersection of planes α and β is perpendicular to the plane γ. ■ |
|
Let us consider a theorem describing the relationship between parallelism and perpendicularity of planes. We already had the corresponding result for straight lines and planes.
Theorem 6 (about parallel planes perpendicular to the third plane).
If one of two parallel planes is perpendicular to the third, then the second plane is perpendicular to it.
Let planes α and β be parallel, and plane γ perpendicular to plane α. Since the plane γ
intersects the plane α, then it must also intersect the plane β parallel to it. Let us take a pro-
an arbitrary straight line m perpendicular to the plane γ and draw through it, as well as through an arbitrary point of the plane β, the plane δ (Fig. 429).
The planes δ and β intersect along a straight line n, and since α ║ β, then m ║ n (Theorem 2 §18). It follows from Theorem 1 that n γ, and therefore the plane β passing through the line n will also be perpendicular to the plane γ.
The proved theorem gives another sign of the perpendicularity of planes.
You can draw a plane perpendicular to the given point through a given point using the sign of perpendicularity of planes (Theorem 1). It is enough to draw a straight line through this point perpendicular to the given plane (see Problem 1 § 19). And then draw a plane through the constructed straight line. It will be perpendicular to the given plane according to the specified criterion. It is clear that an infinite number of such planes can be drawn.
More meaningful is the problem of constructing a plane perpendicular to a given one, provided that it passes through a given line. It is clear that if a given line is perpendicular to a given plane, then an infinite number of such planes can be constructed. It remains to consider the case when the given line is not perpendicular to the given plane. The possibility of such a construction is justified at the level of physical models of straight lines and planes in example 1.
Task 1. Prove that through an arbitrary line not perpendicular to a plane, one can draw a plane perpendicular to the given plane.
Let a plane α and a line l, l B\ a be given. Let us take an arbitrary point M on the line l and draw a line m through it, perpendicular to the plane α (Fig. 430, a). Since, by condition, l is not perpendicular to α, then the lines l and m intersect. Through these straight lines it is possible to draw a plane β (Fig. 430, b), which, according to the test for the perpendicularity of planes (Theorem 1), will be perpendicular to the plane α. ■
Example 3. Through vertex A of the regular pyramid SABC with the base ABC, draw a straight line perpendicular to the plane of the side face of SBC.
To solve this problem, we use the theorem about the perpendicular to the line of intersection of perpendicular planes
(Theorem 2). Let K be the midpoint of edge BC (Fig. 431). The planes AKS and BCS are perpendicular, according to the sign of perpendicularity of planes (Theorem 1). Indeed, BC SK and BC AK are like medians drawn to the bases in isosceles triangles. Therefore, according to the criterion of perpendicularity of a line and a plane (Theorem 1 §18), the line BC is perpendicular to the plane AKS. The BCS plane passes through a line perpendicular to the AKS plane.
Construction. Let us draw a line AL in the AKS plane from point A, perpendicular to the KS line - the line of intersection of the AKS and BCS planes (Fig. 432). By the theorem about the perpendicular to the line of intersection of perpendicular planes (Theorem 2), the line AL is perpendicular to the plane BCS. ■
Control questions |
|||||
In Fig. 433 shows the square ABCD, |
|||||
line MD is perpendicular to the plane |
|||||
ABCD. Which of the pairs of planes are not |
|||||
are perpendicular: |
|||||
MAD and MDC; |
MBC and MAV; |
||||
ABC and MDC; |
MAD and MAV? |
||||
2. In Fig. 434 is shown correctly- new quadrangular pyramid
SABCD, points P, M, N - middle -
The edges AB, BC, BS, O are the center of the base ABCD. Which of the pairs are flat- bones are perpendicular:
1) ACS and BDS; 2) MOS and POS;
3) COS and MNP; 4) MNP and SOB;
5) CND and ABS?
Perpendicularity of lines and planes |
||
3. In Fig. 435 |
depicted rectangular |
|
triangle |
with right angle C and |
|
straight line BP, perpendicular to the plane |
||
ty ABC . Which of the following pairs are flat? |
||
bones are perpendicular: |
||
1) CBP and ABC; |
2) ABP and ABC; |
|
3) PAC and PBC; 4) PAC and PAB?
4. The two planes are perpendicular. Is it possible through an arbitrary point of one of should they draw a straight line in this plane, the second plane?
5. It is impossible to draw a straight line in the α plane, but in the β plane. Could these planes be mi?
6. Through a certain point on the plane α does a line pass in this plane and is perpendicular to the plane, so that the planes α and β are perpendicular?
A section of fence is attached to a vertical post, is it possible to claim that the plane of the fence is vertical?
How to attach a shield vertically to a rail parallel to the surface of the earth?
Why is the surface of the doors, regardless of whether they are closed or open, vertical to the floor?
Why does a plumb line fit tightly against a vertical wall, but not necessarily against an inclined one?
Is it possible to attach a shield to an inclined post so that it is perpendicular to the surface of the earth?
How to practically determine whether a plane is perpendicular
walls plane floor? perpendicularperpendicularperpendicular- straight, lying down - β. True 7. . Possible 8.9.10.11.12.
Graphic exercises
1. In Fig. 436 shows a cube ABCDA 1 B 1 C 1 D 1 .
1) Specify planes perpendicular to the plane VDD 1.
2) How are the planes and
A1 B1 CAB 1 C 1
Perpendicularity of planes |
|||||||
437 plane squares ABCD and |
|||||||
ABC1 D1 |
perpendicular. Distance |
CC1 |
|||||
equals b. Find the length of the segment: |
|||||||
AB; |
D1 C; |
||||||
D1 D; |
C1 D. |
Dan- |
|||||
Construct a drawing according to the given |
|||||||
1) Planes of equilateral triangles |
|||||||
ABC and ABC are perpendicular. |
|||||||
Plane ABC is perpendicular to planes BDC and BEA. |
|||||||
Planes α and β are perpendicular to the plane γ and intersect |
|||||||
along the straight line a, the lines of their intersection with the plane γ |
|||||||
are straight lines b and c. |
|||||||
In a rectangular parallelepiped ABCDA 1 B 1 C 1 D 1 plane |
|||||||
bones AB 1 C 1 and ICA 1 are perpendicular. |
|||||||
421. The segment OS is drawn from the center O of the square ABCD perpendicular to its plane.
1°) Determine the relative position of the ACS planes
and ABC.
2°) Determine the relative position of the ACS planes
and BDS.
3) Construct a plane passing through the straight line OS perpendicular to the ABS plane.
4) Construct a plane perpendicular to plane ABC and passing through the midpoints of sides AD and CD.
422. From the intersection point O of the diagonals of the rhombus ABCD, a segment OS is drawn perpendicular to the plane of the rhombus; AB=DB=
1°) Determine the relative position of the SDB and
ABC, SDB and ACS.
2°) Construct a plane passing through line BC perpendicular to plane ABD.
3) Draw a plane perpendicular to plane ABC through the middle F of segment CS.
4) Find the area of triangle BDF.
423. Given a cube ABCDA1 B1 C1 D1.
1°) Determine the relative position of the planes AB 1 C 1
and CDD1.
2°) Determine the relative position of the planes AB 1 C 1
and CD1 A1.
3°) Construct a plane passing through point A perpendicular to the plane BB 1 D 1.
4) Construct a section of the cube with a plane passing through the midpoints of the edges A 1 D 1 and B 1 C 1 perpendicular to the plane ABC. 5) Determine the relative position of the plane AA 1 B and the plane passing through the middle of the ribs A 1 B 1, C 1 D 1, CD.
6) Find the cross-sectional area of the cube by the plane passing through the edge BB 1 and the middle of the edge A 1 D 1 (BB 1 = a).
7) Construct a point symmetrical to point A relative to the plane A 1 B 1 C.
424. In a regular tetrahedron ABCD with an edge of 2 cm, point M is the midpoint of DB, and point N is the midpoint of AC.
1°) Prove that straight line DB is perpendicular to the plane
2°) Prove that the plane BDM is perpendicular to the plane AMC.
3) Through point O of the intersection of the medians of triangle ADC, draw a straight line perpendicular to the AMC plane.
4) Find the length of this line segment inside the tetrahedron. 5) In what ratio does the AMC plane divide this segment?
425. Two equilateral triangles ABC and ADC lie in perpendicular planes.
1°) Find the length of segment BD if AC = 1 cm.
2) Prove that the plane BKD (K lies on the line AC) is perpendicular to the plane of each of the triangles if and only if K is the midpoint of side AC.
426. Rectangle ABCD, whose sides are 3 cm and 4 cm, is bent along the diagonal AC so that triangles ABC and ADC are located in perpendicular planes. Determine the distance between points B and D after bending the rectangle ABCD.
427. Through this point draw a plane perpendicular to each of the two given planes.
428°. Prove that the planes of adjacent faces of a cube are perpendicular.
429. Planes α and β are perpendicular to each other. From point A of plane α, a straight line AB is drawn perpendicular to plane β. Prove that line AB lies in the α plane.
430. Prove that if a plane and a line not lying in this plane are perpendicular to the same plane, then they are parallel to each other.
431. Through points A and B lying on the line of intersection p of planes α and β perpendicular to each other, straight lines perpendicular to p are drawn: AA 1 in α, BB 1 in β. Point X lies on straight line AA 1, and point Y lies on BB 1. Prove that straight line ВB 1 is perpendicular to straight line ВХ, and straight line АА 1 is perpendicular to straight line АY.
432*. Through the middle of each side of the triangle a plane is drawn perpendicular to this side. Prove that all three drawn planes intersect along one straight line perpendicular to the plane of the triangle.
Exercises to repeat
433. In an equilateral triangle with side b determine: 1) height; 2) radii of the inscribed and circumscribed circles.
434. From one point a perpendicular and two oblique lines are drawn to a given line. Determine the length of the perpendicular if the inclined ones are 41 cm and 50 cm, and their projections onto this line are in the ratio 3:10.
435. Determine the legs of a right triangle if bis- the sectrix of a right angle divides the hypotenuse into segments of 15 cm and
Basic definition
The two planes are called
are perpendicular , if each of them is formed by straight lines- mi, perpendicular- mi of the second plane and passing through the intersection points of these planes.
Main statements |
||||
Perpendicular sign |
If alone |
|||
cularity |
planes |
pass- |
||
planes |
dit through |
|||
perpendicular |
||||
the second plane, then |
b α, b β α β |
|||
these planes are per- |
||||
pendicular. |
||||
perpend- |
two planes |
||||
orifice |
are perpendicular, then |
||||
intersectionsperpen |
direct, belonging to |
||||
dicular |
flat |
sharing one plane |
|||
and perpendicular |
|||||
intersections |
|||||
these planes, per- |
α β, b β, c = α ∩β, |
||||
pendicular to the second |
b c b α |
||||
plane. |
|||||
This article is devoted to perpendicular planes. Definitions and notations will be given along with examples. The sign of perpendicularity of planes and the condition under which it is satisfied will be formulated. Solutions to similar problems will be discussed using examples.
Yandex.RTB R-A-339285-1
If there is an angle between intersecting lines, we can talk about defining perpendicular planes.
Definition 1
Provided that the angle between perpendicular lines is 90 degrees, they are called perpendicular.
The designation of perpendicularity is usually written with the sign “⊥”. If the condition states that the planes α and β are perpendicular, then the entry takes the form α ⊥ β. The picture below shows in detail.
When it is given in the catch that the plane α and β are perpendicular, this means that α is perpendicular to β and vice versa. Such planes are called mutually perpendicular. For example, the wall and ceiling in a room are mutually perpendicular, since when they intersect they form a right angle.
Perpendicularity of planes - sign and condition of perpendicularity
In practice, you can encounter tasks where it is necessary to determine the perpendicularity of given planes. First you need to determine the angle between them. If it is equal to 90 degrees, then they are considered perpendicular from the definition.
To prove the perpendicularity of two planes, the sign of perpendicularity of two planes is used. The formulation contains the concepts of a perpendicular line and a plane. Let us write the exact definition of the perpendicularity criterion in the form of a theorem.
Theorem 1
If one of two given planes intersects a line perpendicular to the other plane, then the given planes are perpendicular.
The proof is available in the geometry textbook for grades 10 - 11, where there is a detailed description. It follows from the sign that if a plane is perpendicular to the line of intersection of two given planes, then it is perpendicular to each of these planes.
There is a necessary and sufficient condition for proof. Let's consider them for the perpendicularity of two given planes, which is used as a check of their perpendicularity, located in a rectangular coordinate system of three-dimensional space. For the proof to be valid, it is necessary to apply the definition of the normal vector of a plane, which helps to prove the necessary and sufficient condition for the perpendicularity of planes.
Theorem 2
In order for the perpendicularity of intersecting planes to be obvious, it is necessary and sufficient that the normal vectors of the given planes intersect at right angles.
Proof
Let a rectangular coordinate system be specified in three-dimensional space. If we have n 1 → = (A 1, B 1, C 1) and n 2 → = (A 2, B 2, C 2), which are normal vectors of the given planes α and β, then a necessary and sufficient condition for the perpendicularity of the vectors n 1 → and n 2 → will take the form
n 1 → , n 2 → = 0 ⇔ A 1 · A 2 + B 1 · B 2 + C 1 · C 2 = 0
From here we get that n 1 → = (A 1, B 1, C 1) and n 2 → = (A 2, B 2, C 2) are normal vectors of given planes, and for the reality of perpendicularity of α and β it is necessary and sufficient, so that the scalar product of the vectors n 1 → and n 2 → is equal to zero, and therefore takes the form n 1 → , n 2 → = 0 ⇔ A 1 · A 2 + B 1 · B 2 + C 1 · C 2 = 0 .
The equality is fulfilled.
Let's take a closer look at examples.
Example 1
Determine the perpendicularity of the planes specified in the rectangular coordinate system O x y z of the three-dimensional space specified by the equations x - 3 y - 4 = 0 and x 2 3 + y - 2 + z 4 5 = 1?
Solution
To find the answer to the question of perpendicularity, you first need to find the coordinates of the normal vectors of the given planes, after which you can check for perpendicularity.
x - 3 y - 4 = 0 is a general equation of the plane, from which you can immediately transform the coordinates of the normal vector, equal to n 1 → = (1, - 3, 0).
To determine the coordinate of the normal vector of the plane x 2 3 + y - 2 + z 4 5 = 1, let's move from the equation of the plane in segments to the general one.
Then we get:
x 2 3 + y - 2 + z 4 5 ⇔ 3 2 x - 1 2 y + 5 4 z - 1 = 0
Then n 2 → = 3 2, - 1 2, 5 4 are the coordinates of the normal vector of the plane x 2 3 + y - 2 + z 4 5 = 1.
Let's move on to calculating the scalar product of vectors n 1 → = (1, - 3, 0) and n 2 → = 3 2, - 1 2, 5 4.
We obtain that n 1 → , n 2 → = 1 · 3 2 + (- 3) · - 1 2 + 0 · 5 4 = 3 .
We see that it is not equal to zero, which means that the given vectors are not perpendicular. It follows that the planes are also not perpendicular. The condition is not met.
Answer: planes are not perpendicular.
Example 2
The rectangular coordinate system O x y z has four points with coordinates A - 15 4, - 7 8, 1, B 17 8, 5 16, 0, C 0, 0, 3 7, D - 1, 0, 0. Check whether planes A B C and A B D are perpendicular.
Solution
First, you need to calculate the scalar product of the vectors of these planes. If it is equal to zero, only in this case can we consider that they are perpendicular. We find the coordinates of the normal vectors n 1 → and n 2 → planes A B C and A B D.
From the given coordinates of the points, we calculate the coordinates of the vectors A B → , A C → , A D → . We get that:
A B → = 47 8, 19 16, - 1, A C → = 15 4, 7 8, - 4 7, A D → = 11 4, 7 8, - 1.
The normal vector of the plane A B C is the vector product of the vectors A B → and A C →, and for A B D the vector product of A B → and A D →. From here we get that
n 1 → = A B → × A C → = i → j → k → 47 8 19 16 - 1 15 4 7 8 - 4 7 = 11 56 i → - 11 28 j → + 11 16 k → ⇔ n 1 → = 11 56 , - 11 28 , 11 16 n 2 → = A B → × A D → = i → j → k → 47 8 19 16 - 1 11 4 7 8 - 1 = - 5 16 i → + 25 8 j → + 15 8 k → ⇔ n 2 → = - 5 16 , 25 8 , 15 8
Let's start finding the scalar product n 1 → = 11 56, - 11 28, 11 16 and n 2 → = - 5 16, 25 8, 15 8.
We get: n 1 → , n 2 → = 11 56 · - 5 16 + - 11 28 · 25 8 + 11 16 · 15 8 = 0 .
If it is equal to zero, then the vectors of the planes A B C and A B D are perpendicular, then the planes themselves are perpendicular.
Answer: planes are perpendicular.
It was possible to approach the solution differently and use the equations of the planes A B C and A B D. After finding the coordinates of the normal vectors of these planes, it would be possible to check whether the condition of perpendicularity of the normal vectors of the planes is satisfied.
If you notice an error in the text, please highlight it and press Ctrl+Enter
